Here’s a great challenge for my readers: Robert Bunch (1820-1881), a British spy in the US Civil War, left behind a number of encrypted messages that have never been deciphered. Can you solve this mystery?
Robert Bunch (1820-1881) was a British diplomat, who worked as a secret agent in Charleston, South Carolina, during the Civil War. Officially being a British representative following commercial matters, Bunch’s secret job was to inform his government about what was going on in the secessionist South of the US during the war. The British crown faced the dilemma that, on the one hand, they needed the cheap cotton they received from Southern State producers, while, on the other hand, they opposed the slave holding practices the US cotton production was built on.
The British even considered to mediate between the North and the South in the Civil War, but based on Bunch’s reports they realized that a solution acceptable for all parties was out of reach.
The story of Robert Bunch is told in the book Our Man in Charleston written by US author Christopher Dickey. An interview with Dickey about this work is available here.
Bunch’s encrypted notes
A few days ago, I received an email from Colin Beveridge, who had listened to my recent talk Solving Historical Ciphers with Modern Means at the History of Cryptography and Codes in London (organized by the British Society for the History of Mathematics). Colin has a very interesting website titled Flying Colours Maths. If you are looking for easy-to-unterstand information about mathematics, this is the place to go.
Colin wrote me because he had been contacted by afore-mentioned Christopher Dickey. When researching the life of Robert Bunch, Dickey encountered a number of encrypted notes Bunch had left behind. Apparently, nobody has been able to decipher it, so far.
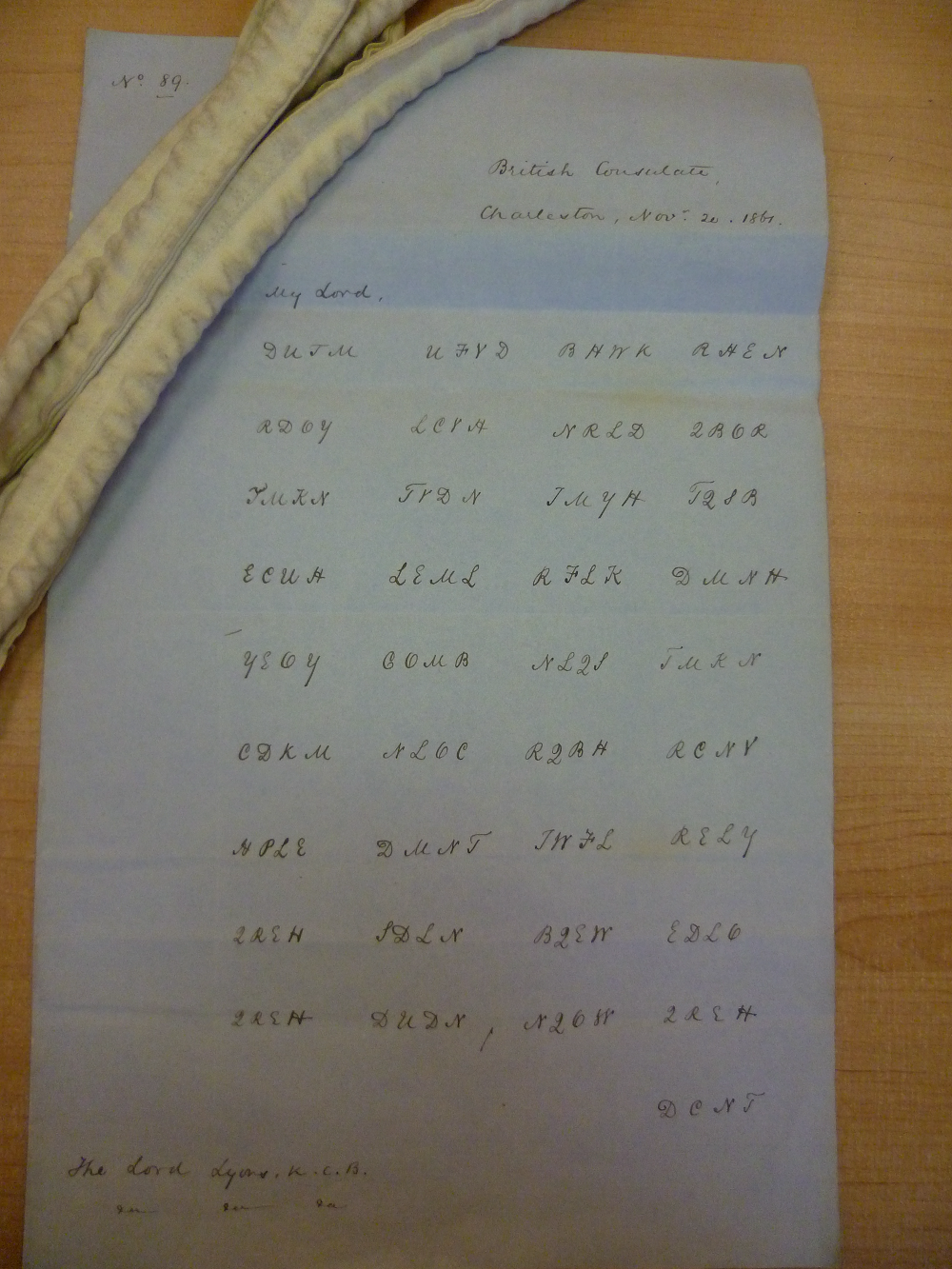
Colin has provided me five cryptogram scans. Here they are (with transcriptions Colin made):
#1
#2
EOCH TQOL BTOU QREH DVTM NRLF DUTN DRSK MYHT DMNT QKYD BYCV PDUR BOHK RYEL TLOT BDVH BQRY RYBK POFW DELY SEPT LKOM TMKN XREH HWTY NLHZ CPQV BDUH
#3
DUTM CBFV RQBH MEOT NKMQ NDMO SFWE HLEK RCUW QREH DMNT RBUE EKRS QREH CWRV EQTY SEPD MNLE ELLU TMKN WBEP RISD GMOS RLEL
#4
DUTM UFVD BHWK RHEN RDOY LCVH NRLD QBOR TMKN TVDN TMYH TQSB ECUH LEML RFLK DMNH YEOY COMB NLQS TMKN CDKM NLOC RQBH RCNV HPLE DMNT TWFL RELY QREH SDLN BQEW EDLO QREH DUDN NQOW QREH DCNT
#5
Can you master the cypher?
The following letter is not encrypted but the author states that he has “mastered the cypher”.
Now, the question is whether a reader of this blog can master these encrypted messages, too. Encrypted messages from the US Civil War are not uncommon, as encryption played an important role in this war. The ciphers used back then were not the best, so virtually all known cryptograms from the Civil War have been deciphered. This one is probably more advanced than most other Civil War ciphers (I suppose that the British had better encryption methods than the US at that time), but it’s probably not unsolvable. My guess is that a polyalphabetical cipher (perhaps, a Vigenère variant) was used.
Can a reader find out more? If so, please let Christopher Dickey, Colin Beveridge and me know.
Follow @KlausSchmeh
Further reading: Who can decipher this Pitman letter from the US Civil War?
Linkedin: https://www.linkedin.com/groups/13501820
Facebook: https://www.facebook.com/groups/763282653806483/

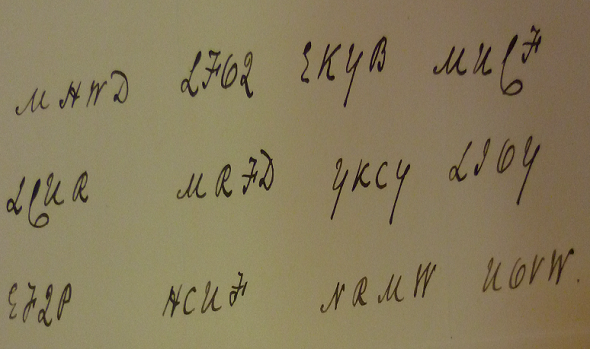






Kommentare (26)